Topology is a branch of mathematics that deals with the study of limits and the continuity of functions. Together with algebra, topology constitutes the general basis of mathematics.
Topological space or figure - a subset of our homogeneous Euclidean space, between the points of which some proximity relation is given. Here the figures are considered not as rigid bodies, but as objects made, as it were, of very elastic rubber, allowing continuous deformation, preserving their qualitative properties.
A one-to-one continuous mapping of figures is called homeomorphism. In other words, the figures homeomorphic, if one can be converted into another by continuous deformation.
Examples. The following figures are homeomorphic (figures from different groups are not homeomorphic), shown in Fig. 2.
1. Segment and curve without self-intersections.
2. Circle, square inside, tape.
3. Sphere, cube and tetrahedron surface.
4. Circle, ellipse and knotted circle.
5. A ring on a plane (a circle with a hole), a ring in space, a ring twisted twice, the side surface of a cylinder.
6. Mobius strip, i.e. once twisted ring, and three times twisted ring.
7. The surface of a torus (donut), a sphere with a handle, and a knotted torus.
8. Sphere with two handles and a pretzel with two holes.
In mathematical analysis, functions are studied by the method of limits. Variable and limit are the basic concepts.
In various phenomena, some quantities retain their numerical value, others change. The set of all numerical values of a variable is called the scope of this variable.
Of the various ways in which a variable behaves, the most important is the one in which the variable tends to a certain limit.
constant number a called variable x if the absolute value of the difference between x and a(
) becomes in the process of changing the variable x arbitrarily small: 
What does "arbitrarily small" mean? variable X tends to the limit a, if for any arbitrarily small (arbitrarily small) number there is such a moment in the change of the variable X, starting from which the inequality 
.
The definition of a limit has a simple geometric meaning: the inequality 
means that X is in -neighborhood of the point a,
those. in the interval 
.
Thus, the definition of the limit can be given in geometric form:
Number a is the limit of the variable X, if for any arbitrarily small (arbitrarily small) -neighborhood of the number a you can specify such a moment in changing the variable X, starting from which all its values fall into the specified -neighborhood of the point a.
Comment. variable X can approach its limit in different ways: remaining less than this limit (on the left), more (on the right), fluctuating around the value of the limit.
Sequence limit
Function called the law (rule) according to which each element x some set X matches a single element y sets Y.
The function can be defined on the set of all natural numbers: . Such a function is called natural argument function or numerical sequence.
Since the sequence, like any infinite set, cannot be specified by enumeration, it is specified by a common member: 
, where is the common term of the sequence.
A discrete variable is a common member of a sequence.
For a sequence, the words "starting at some point" mean the words "starting at some number."
Number a is called the limit of the sequence 
, if for any arbitrarily small (arbitrarily small) number there exists such a number N, which for all members of the sequence with number n>N the inequality 
.
or 
at 
.
Geometrically, the definition of the limit of a sequence means the following: for any arbitrarily small (arbitrarily small) -neighborhood of a number a there is a number such that all terms of the sequence with greater than N, numbers, fall into this neighborhood. Outside the neighborhood is only a finite number of initial terms of the sequence. Natural number N depends on : 
.
VARIABLES AND CONSTANTS
As a result of measuring physical quantities (time, area, volume, mass, speed, etc.), their numerical values. Mathematics deals with quantities, abstracting from their concrete content. In what follows, when speaking of quantities, we will mean their numerical values. In various phenomena, some quantities change, while others retain their numerical value. For example, when a point moves uniformly, time and distance change, but the speed remains constant.
variable A quantity that takes on various numerical values is called. A quantity whose numerical values do not change is called constant. Variable quantities will be denoted by letters x, y, z,…, constant - a, b, c,…
Note that in mathematics a constant value is often considered as a special case of a variable in which all numerical values are the same.
Change area A variable is the totality of all the numerical values that it accepts. The area of change can consist of one or more intervals, or of a single point.
ORDERED VARIABLE. NUMERICAL SEQUENCE
We will say that the variable x there is ordered variable, if the area of its change is known, and for each of any two of its values it is possible to say which of them is the previous and which is the next.
A special case of an ordered variable is a variable whose values form number sequence x 1 ,x 2 ,…,x n ,… For such values at i< j, i, j Î N , meaning x i considered to be prior, xj– subsequent, regardless of which of these values is greater. Thus, a numerical sequence is a variable whose successive values can be renumbered. The numerical sequence will be denoted by . The individual numbers of a sequence are called its elements.
For example, the numerical sequence is formed by the following quantities:
FUNCTION
When studying various natural phenomena and solving technical problems, and, consequently, in mathematics, one has to consider the change in one quantity depending on the change in another. So, for example, it is known that the area of a circle is expressed in terms of the radius by the formula S = pr2. If the radius r takes on different numerical values, then the area S also takes on various numeric values, i.e. a change in one variable entails a change in the other.
If each value of the variable x, belonging to some area, corresponds to one specific value of another variable y, then y called function of variable x. We will symbolically write y=f(x). At the same time, the variable x called independent variable or argument.
Recording y=C, where C- constant, denotes a function, the value of which, for any value x the same and equal C.
Many values x, for which it is possible to determine the values of the function y according to the rule f(x), is called function scope.
Note that a numerical sequence is also a function whose domain of definition coincides with the set of natural numbers.
The main elementary functions include all the functions studied in the school mathematics course:
elementary function is a function that can be given by basic elementary functions and constants using a finite number of operations of addition, subtraction, multiplication, division, and taking a function from a function.
THE CONCEPT OF THE LIMIT OF A NUMERICAL SEQUENCE
In the further course of mathematics, the concept of the limit will play a fundamental role, since the basic concepts are directly related to it. mathematical analysis- derivative, integral, etc.
Let's start with the concept of the limit of a numerical sequence.
Number a called limit sequences x = {x n) if for an arbitrary preassigned arbitrarily small positive number ε there exists a natural number N, that for all n>N the inequality |x n - a|< ε.
If number a there is a sequence limit x = {x n), then they say that x n tends to a, and write .
To formulate this definition in geometric terms, we introduce the following notion.
Neighborhood of point x 0 is called an arbitrary interval ( a, b) containing this point inside itself. The neighborhood of a point is often considered x0, for which x0 is the middle, then x0 called center neighborhood, and the quantity ( b–a)/2 – radius neighborhood.
So, let's find out what the concept of the limit of a numerical sequence means geometrically. To do this, we write the last inequality from the definition in the form
This inequality means that all elements of the sequence with numbers n>N must lie in the interval (a – ε; a + ε).
Therefore, a constant number a is the limit of the numerical sequence ( x n) if for any small neighborhood centered at a point a radius ε (ε are neighborhoods of the point a) there is such an element of the sequence with number N that all subsequent elements with numbers n>N will be within this neighborhood.
Examples.
Let's make some remarks.
Remark 1. Obviously, if all elements of a numerical sequence take on the same constant value x n = c, then the limit of this sequence will be equal to the constant itself. Indeed, for any ε the inequality | xn-c| = |c - c| = 0 < ε.
Remark 2. It follows from the definition of a limit that a sequence cannot have two limits. Indeed, suppose that x n → a and at the same time x n → b. Take any and mark the neighborhoods of the points a and b radius ε (see Fig.). Then, by the definition of the limit, all elements of the sequence, starting from some, must be located both in the neighborhood of the point a, and in the vicinity of the point b, which is impossible.
Remark 3. It should not be thought that every numerical sequence has a limit. Let, for example, the variable takes the values 
. It is easy to see that this sequence does not tend to any limit.
FUNCTION LIMIT
Let the function y=f(x) defined in some neighborhood of the point a. Let's assume that the independent variable x approaches indefinitely a. This means that we can give X values arbitrarily close to a but not equal a. Let's denote it like this x → a. For such x find the corresponding values of the function. It may happen that the values f(x) also indefinitely approach some number b.Then they say that the number b there is a function limit f(x) at x → a.
Let us introduce a rigorous definition of the limit of a function.
Function y=f(x) tends to the limit b as x → a, if for each positive number ε, no matter how small it is, one can specify a positive number δ such that for all x ≠ a from the domain of the function that satisfy the inequality | x-a| < δ, имеет место неравенство |f(x) - b| < ε. Если b there is a function limit f(x) at x → a, then write or f(x) → b at x → a.
We illustrate this definition on the graph of the function. Because from inequality | x-a| < δ должно следовать неравенство |f(x) - b| < ε, т.е. при x Î ( a - δ, a+ δ) the corresponding values of the function f(x) Î ( b - ε, b+ ε), then, taking an arbitrary ε > 0, we can choose a number δ such that for all points x, lying in δ, a neighborhood of the point a, the corresponding points of the graph of the function must lie inside a strip of width 2ε bounded by straight lines y=b– ε and y=b + ε.
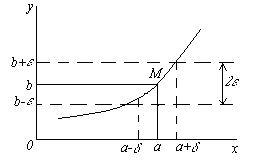
It is easy to see that the limit of a function must have the same properties as the limit of a numerical sequence, namely, if for x → a function has a limit, then it is unique.
Examples.
THE CONCEPT OF THE LIMIT OF A FUNCTION AT A POINT OF INTERNITY
So far, we have considered limits for the case when the variable x strived for a certain constant number.
We will say that the variable x tends to infinity, if for each predetermined positive number M(it can be arbitrarily large) you can specify such a value x=x 0, starting from which, all subsequent values of the variable will satisfy the inequality |x|>M.
For example, let the variable X takes values x 1 = –1, x 2 = 2, x 3 = –3, …, x n=(–1) n n, … It is clear that this is an infinitely large variable, since for all M> 0, all values of the variable, starting from some, will be greater in absolute value M.
variable x → +∞, if for an arbitrary M> 0, all subsequent values of the variable, starting from a certain one, satisfy the inequality x > M.
Likewise, x→ – ∞, if for any M > 0 x< -M .
We will say that the function f(x) tends to the limit b at x→ ∞ if for an arbitrary small positive number ε one can find such a positive number M, which for all values x satisfying the inequality |x|>M, the inequality | f(x) - b| < ε.
Designate .
Examples.
INFINITELY BIG FEATURES
Earlier we considered cases where the function f(x) striving for some final limit b at x → a or x → ∞.
Consider now the case when the function y=f(x) some way to change the argument.
Function f(x) tends to infinity as x → a, i.e. is an infinitely large value, if for any number M, however large it may be, one can find δ > 0 such that for all values X≠a, satisfying the condition | x-a| < δ, имеет место неравенство |f(x)| > M.
If f(x) tends to infinity as x→a, then write or f(x)→∞ at x→a.
Formulate a similar definition for the case when x→∞.
If f(x) tends to infinity as x→a and at the same time takes only positive or only negative values, respectively write or .
Examples.
LIMITED FUNCTIONS
Let the function y=f(x), defined on some set D argument values.
Function y=f(x) called limited on the set D if there is a positive number M such that for all values x from the set under consideration, the inequality |f(x)|≤M. If such a number M does not exist, then the function f(x) called unlimited on the set D.
Examples.
- Function y=sin x, defined at -∞<x<+∞, является ограниченной, так как при всех значениях x|sin x|≤1 = M.
- Function y=x 2 +2 is limited, for example, on the interval , since for all x from this segment |f(x)| ≤f(3) = 11.
- Consider the function y=ln x at xн (0; 1). This function is unbounded on the indicated segment, since for x→0 log x→-∞.
Function y=f(x) called bounded as x → a if there is a neighborhood centered at a point a where the function is limited.
Function y=f(x) called bounded as x→∞ if there is such a number N> 0, which for all values X |x|>N, function f(x) limited.
Let us establish a connection between a bounded function and a function that has a limit.
Theorem 1. If b is a finite number, then the function f(x) limited at x→a.
Proof. Because , then for any ε>0 there is a number δ>0 such that for all values X satisfying the inequality |x-a|<
δ, the inequality |f(x) –b|<
ε. Using the module property |f(x) – b|≥|f(x)| - |b|, we write the last inequality in the form |f(x)|<|b|+
ε. Thus, if we put M=|b|+ε, then x→a |f(x)|
Comment. It follows from the definition of a bounded function that if , then it is unbounded. However, the converse is not true: an unbounded function may not be infinitely large. Give an example.
Theorem 2. If , then the function y=1/f(x) limited at x→a.
Proof. It follows from the conditions of the theorem that, for arbitrary ε>0, in some neighborhood of the point a we have |f(x) – b|<
ε. Because |f(x) – b|=|b – f(x)| ≥|b| - |f(x)|, then |b| - |f(x)|<
ε. Hence, |f(x)|>|b| -ε >0. That's why 
The concept of the limit of a numerical sequence
Let us first recall the definition of a numerical sequence.
Definition 1
Mappings of the set of natural numbers onto the set real numbers called numerical sequence.
The concept of the limit of a numerical sequence has several basic definitions:
- A real number $a$ is called the limit of a numerical sequence $(x_n)$ if for any $\varepsilon >0$ there exists an index $N$ depending on $\varepsilon$ such that for any index $n> N$ the inequality $\left|x_n-a\right|
- A real number $a$ is called the limit of a numerical sequence $(x_n)$ if any neighborhood of the point $a$ contains all the members of the sequence $(x_n)$, with the possible exception of a finite number of members.
Consider an example of calculating the value of the limit of a numerical sequence:
Example 1
Find the limit $(\mathop(lim)_(n\to \infty ) \frac(n^2-3n+2)(2n^2-n-1)\ )$
Solution:
To solve this task, we first need to take out the parentheses of the highest degree included in the expression:
$(\mathop(lim)_(n\to \infty ) \frac(n^2-3n+2)(2n^2-n-1)\ )=(\mathop(lim)_(x\to \ infty ) \frac(n^2\left(1-\frac(3)(n)+\frac(2)(n^2)\right))(n^2\left(2-\frac(1) (n)-\frac(1)(n^2)\right))\ )=(\mathop(lim)_(n\to \infty ) \frac(1-\frac(3)(n)+\ frac(2)(n^2))(2-\frac(1)(n)-\frac(1)(n^2))\ )$
If the denominator is an infinitely large value, then the entire limit tends to zero, $\mathop(lim)_(n\to \infty )\frac(1)(n)=0$, using this, we get:
$(\mathop(lim)_(n\to \infty ) \frac(1-\frac(3)(n)+\frac(2)(n^2))(2-\frac(1)(n )-\frac(1)(n^2))\ )=\frac(1-0+0)(2-0-0)=\frac(1)(2)$
Answer:$\frac(1)(2)$.
The concept of the limit of a function at a point
The concept of the limit of a function at a point has two classical definitions:
Definition of the term "limit" according to Cauchy
A real number $A$ is called the limit of the function $f\left(x\right)$ as $x\to a$ if for any $\varepsilon > 0$ there exists $\delta >0$ depending on $\varepsilon $, such that for any $x\in X^(\backslash a)$ satisfying the inequality $\left|xa\right|
Heine definition
A real number $A$ is called the limit of the function $f\left(x\right)$ for $x\to a$ if for any sequence $(x_n)\in X$ converging to $a$ the sequence of values $f (x_n)$ converges to $A$.
These two definitions are related.
Remark 1
The Cauchy and Heine definitions of the limit of a function are equivalent.
In addition to the classical approaches to calculating the limits of a function, let's recall formulas that can also help in this.
Table of equivalent functions when $x$ is infinitesimal (goes to zero)
One approach to solving limits is principle of replacement by an equivalent function. The table of equivalent functions is presented below, in order to use it, instead of the functions on the right, substitute the corresponding elementary function on the left into the expression.
Figure 1. Function equivalence table. Author24 - online exchange of student papers
Also, to solve the limits, the values of which are reduced to uncertainty, it is possible to apply the L'Hospital rule. In the general case, the uncertainty of the form $\frac(0)(0)$ can be revealed by factoring the numerator and denominator and then reducing. An indeterminacy of the form $\frac(\infty )(\infty)$ can be resolved after dividing the expressions in the numerator and denominator by the variable at which the highest power is found.
Remarkable Limits
- First remarkable limit:
$(\mathop(lim)_(x\to 0) \frac(sinx)(x)\ )=1$
- Second remarkable limit:
$\mathop(lim)_(x\to 0)((1+x))^(\frac(1)(x))=e$
Special Limits
- First special limit:
$\mathop(lim)_(x\to 0)\frac(((log)_a (1+x-)\ ))(x)=((log)_a e\ )=\frac(1)(lna )$
- Second special limit:
$\mathop(lim)_(x\to 0)\frac(a^x-1)(x)=lna$
- Third special limit:
$\mathop(lim)_(x\to 0)\frac(((1+x))^(\mu )-1)(x)=\mu $
Function continuity
Definition 2
A function $f(x)$ is called continuous at a point $x=x_0$ if $\forall \varepsilon >(\rm 0)$ $\exists \delta (\varepsilon ,E_(0))>(\rm 0) $ such that $\left|f(x)-f(x_(0))\right|
The function $f(x)$ is continuous at the point $x=x_0$ if $\mathop((\rm lim\; ))\limits_((\rm x)\to (\rm x)_((\rm 0 )) f(x)=f(x_(0))$.
A point $x_0\in X$ is called a discontinuity point of the first kind if it has finite limits $(\mathop(lim)_(x\to x_0-0) f(x_0)\ )$, $(\mathop(lim) _(x\to x_0+0) f(x_0)\ )$, but $(\mathop(lim)_(x\to x_0-0) f(x_0)\ )=(\mathop(lim)_ (x\to x_0+0) f(x_0)\ )=f(x_0)$
Moreover, if $(\mathop(lim)_(x\to x_0-0) f(x_0)\ )=(\mathop(lim)_(x\to x_0+0) f(x_0)\ )\ne f (x_0)$, then this is a break point, and if $(\mathop(lim)_(x\to x_0-0) f(x_0)\ )\ne (\mathop(lim)_(x\to x_0+ 0) f(x_0)\ )$, then the jump point of the function.
A point $x_0\in X$ is called a discontinuity point of the second kind if it contains at least one of the limits $(\mathop(lim)_(x\to x_0-0) f(x_0)\ )$, $(\mathop( lim)_(x\to x_0+0) f(x_0)\ )$ represents infinity or does not exist.
Example 2
Investigate for continuity $y=\frac(2)(x)$
Solution:
$(\mathop(lim)_(x\to 0-0) f(x)\ )=(\mathop(lim)_(x\to 0-0) \frac(2)(x)\ )=- \infty $ - the function has a break point of the second kind.
Function continuity. Break points.
A bull is walking, swinging, sighing on the go:
- Oh, the board is ending, now I will fall!
In this lesson, we will analyze the concept of continuity of a function, the classification of discontinuity points, and a common practical problem investigation of a function for continuity. From the very title of the topic, many intuitively guess what will be discussed, and think that the material is quite simple. It's true. But it is simple tasks that are most often punished for neglect and a superficial approach to solving them. Therefore, I recommend that you carefully study the article and catch all the subtleties and techniques.
What do you need to know and be able to do? Not very much. For a good learning experience, you need to understand what function limit. For readers with a low level of preparation, it is enough to comprehend the article Limits of functions. Solution examples and see the geometric meaning of the limit in the manual Graphs and properties of elementary functions. It is also advisable to familiarize yourself with geometric transformations of graphs, since practice in most cases involves the construction of a drawing. The prospects are optimistic for everyone, and even a full kettle will be able to cope with the task on its own in the next hour or two!
Function continuity. Breakpoints and their classification
The concept of continuity of a function
Consider some function continuous on the entire real line: 
Or, more concisely, our function is continuous on (the set of real numbers).
What is the "philistine" criterion of continuity? It is obvious that the graph of a continuous function can be drawn without lifting the pencil from the paper.
At the same time, it is important to distinguish between two simple concepts: function scope and function continuity. In general it's not the same. For instance: 
This function is defined on the entire number line, that is, for everyone the value of "x" has its own value of "y". In particular, if , then . Note that the other dot is punched out, because by definition of the function, the value of the argument must match the only thing function value. In this way, domain our features: .
but this function is not continuous on ! It is quite obvious that at the point she endures gap. The term is also quite intelligible and clear, indeed, here the pencil will have to be torn off the paper anyway. A little later, we will consider the classification of breakpoints.
Continuity of a function at a point and on an interval
In a particular mathematical problem, we can talk about the continuity of a function at a point, the continuity of a function on an interval, half-interval, or the continuity of a function on a segment. That is, there is no "just continuity"– the function can be continuous SOMEWHERE. And the fundamental "brick" of everything else is function continuity at the point .
The theory of mathematical analysis defines the continuity of a function at a point with the help of "delta" and "epsilon" neighborhoods, but in practice another definition is in use, to which we will pay close attention.
Let's remember first unilateral limits who burst into our lives at the first lesson about function graphs. Consider a daily situation: 
If we approach along the axis to the point left(red arrow), then the corresponding values \u200b\u200bof the "games" will go along the axis to the point (raspberry arrow). Mathematically, this fact is fixed using left-hand limit:
Pay attention to the entry (it reads "x tends to ka from the left"). "Additive" "minus zero" symbolizes , which essentially means that we are approaching the number from the left side.
Similarly, if you approach the point "ka" on right(blue arrow), then the “games” will come to the same value , but along the green arrow, and right-hand limit will be formatted as follows:
"Supplement" symbolizes , and the entry reads like this: "x tends to ka from the right."
If one-sided limits are finite and equal(as in our case): 
, then we will say that there is a GENERAL limit . It's simple, the total limit is our "usual" function limit equal to the final number.
Note that if the function is not defined at (punch out the black dot on the graph branch), then the listed calculations remain valid. As has been repeatedly noted, in particular in the article about infinitesimal functions, expressions mean that "x" infinitely close approaches the point , while IRRELEVANT whether the function itself is defined at the given point or not. A good example will be found in the next section, when the function is analyzed.
Definition: a function is continuous at a point if the limit of the function at a given point is equal to the value of the function at that point: .
The definition is detailed in the following terms:
1) The function must be defined at the point , that is, the value must exist.
2) There must be a common limit of the function . As noted above, this implies the existence and equality of one-sided limits: 
.
3) The limit of the function at a given point must be equal to the value of the function at this point: .
If violated at least one of the three conditions, then the function loses the property of continuity at the point .
Continuity of a function on an interval formulated witty and very simply: a function is continuous on an interval if it is continuous at every point of the given interval.
In particular, many functions are continuous on the infinite interval, that is, on the set of real numbers. This is a linear function, polynomials, exponent, sine, cosine, etc. And in general, any elementary function continuous on its domains, so, for example, the logarithmic function is continuous on the interval . I hope by now you have a good idea of what the graphs of the main functions look like. More detailed information about their continuity can be obtained from a kind man named Fichtenholtz.
With the continuity of the function on the segment and half-intervals, everything is also simple, but it is more appropriate to talk about this in the lesson on finding the minimum and maximum values of a function on a segment until then, let's keep our heads down.
Classification of break points
The fascinating life of the functions is rich in all sorts of special points, and the breaking points are just one of the pages of their biography.
Note : just in case, I will dwell on an elementary moment: the breaking point is always single point- there is no "several break points in a row", that is, there is no such thing as a "break interval".
These points, in turn, are divided into two large groups: breaks of the first kind and breaks of the second kind. Each type of break has its own characteristics which we will look at right now:
Discontinuity point of the first kind
If the continuity condition is violated at a point and unilateral limits finite , then it is called breaking point of the first kind.
Let's start with the most optimistic case. According to the initial idea of the lesson, I wanted to tell the theory “in general terms”, but in order to demonstrate the reality of the material, I settled on a variant with specific actors.
Sadly, like a photo of the newlyweds against the backdrop of the Eternal Flame, but the following frame is generally accepted. Let's draw a graph of the function in the drawing: 
This function is continuous on the entire number line, except for the point. Indeed, the denominator cannot be equal to zero. However, in accordance with the meaning of the limit - we can infinitely close approach “zero” both from the left and from the right, that is, one-sided limits exist and, obviously, coincide: 
(Continuity condition No. 2 is met).
But the function is not defined at the point , therefore, Condition No. 1 of continuity is violated, and the function suffers a break at this point.
A break of this kind (with the existing general limit) are called repairable gap. Why removable? Because the function can redefine at the breaking point: 
Does it look strange? Maybe. But such a function record does not contradict anything! Now the gap is fixed and everyone is happy: 
Let's do a formal check:
2) 
– there is a common limit;
3)
Thus, all three conditions are satisfied, and the function is continuous at a point by the definition of continuity of a function at a point.
However, matan haters can redefine the function in a bad way, for example 
:
Curiously, the first two continuity conditions are satisfied here:
1) - the function is defined at a given point;
2) 
– there is a common limit.
But the third boundary has not been passed: , that is, the limit of the function at the point not equal the value of the given function at the given point.
Thus, at a point, the function suffers a discontinuity.
The second, sadder case is called break of the first kind with a jump. And sadness is evoked by one-sided limits that finite and different. An example is shown in the second drawing of the lesson. This gap usually occurs in piecewise functions already mentioned in the article. about chart transformations.
Consider a piecewise function 
and execute her drawing. How to build a graph? Very simple. On the half-interval we draw a fragment of the parabola (green), on the interval - a straight line segment (red), and on the half-interval - a straight line (blue).
At the same time, due to inequality, the value is defined for a quadratic function (green dot), and due to inequality, the value is defined for a linear function (blue dot): 
In the most difficult case, one should resort to pointwise construction of each piece of the graph (see the first lesson about graphs of functions).
For now, we are only interested in the point . Let's examine it for continuity:
2) Calculate one-sided limits.
On the left we have a red line segment, so the left-hand limit is: 
On the right is the blue straight line, and the right-hand limit: 
As a result, finite numbers, and they not equal. Because one-sided limits finite and different: 
, then our function suffers discontinuity of the first kind with a jump.
It is logical that the gap cannot be eliminated - the function cannot really be further defined and “not glued together”, as in the previous example.
Discontinuity points of the second kind
Usually, all other cases of rupture are cunningly attributed to this category. I will not list everything, because in practice in 99% of tasks you will encounter endless gap- when left-handed or right-handed, and more often, both limits are infinite.
And, of course, the most obvious picture is a hyperbole at zero. Here both one-sided limits are infinite: 
, therefore, the function suffers a discontinuity of the second kind at the point .
I try to fill my articles with the most diverse content, so let's look at the graph of the function, which has not yet been seen: 
according to the standard scheme:
1) The function is not defined at this point because the denominator goes to zero.
Of course, one can immediately conclude that the function suffers a break at the point , but it would be nice to classify the nature of the break, which is often required by condition. For this:
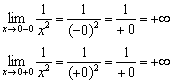
I remind you that a record means infinitesimal negative number, and under the entry - infinitesimal positive number.
The one-sided limits are infinite, which means that the function suffers a discontinuity of the 2nd kind at the point . The y-axis is vertical asymptote for the chart.
It is not rare that both one-sided limits exist, but only one of them is infinite, for example: 
This is the graph of the function.
We examine the point for continuity:
1) The function is not defined at this point.
2) Calculate one-sided limits: 
We will talk about the methodology for calculating such one-sided limits in the last two examples of the lecture, although many readers have already seen and guessed everything.
The left-hand limit is finite and equal to zero (we “do not go to the point itself”), but the right-hand limit is infinite and the orange branch of the graph is infinitely close to its own vertical asymptote, given by the equation(black dotted line).
Thus, the function suffers break of the second kind at point .
As for a discontinuity of the 1st kind, a function can be defined at the discontinuity point itself. For example, for a piecewise function 
boldly put a black bold dot at the origin. On the right is a branch of the hyperbola, and the right-hand limit is infinite. I think almost everyone imagined what this graph looks like.
What everyone was looking forward to:
How to investigate a function for continuity?
The study of the function for continuity at a point is carried out according to the already rolled routine scheme, which consists in checking three continuity conditions:
Example 1
Explore Function 
Solution:
1) The only point falls under the sight, where the function is not defined.
2) Calculate one-sided limits: 
One-sided limits are finite and equal.
Thus, at a point, the function suffers a discontinuable discontinuity.
What does the graph of this function look like?
I want to simplify 
, and it seems to be an ordinary parabola. BUT the original function is not defined at point , so the following caveat is required:
Let's execute the drawing: 
Answer: the function is continuous on the entire number line except for the point where it suffers a discontinuity.
The function can be redefined in a good or not so good way, but this is not required by the condition.
You say the example is far-fetched? Not at all. Happened dozens of times in practice. Almost all tasks of the site come from real independent and control work.
Let's break down our favorite modules:
Example 2
Explore Function 
for continuity. Determine the nature of function breaks, if any. Execute the drawing.
Solution: for some reason, students are afraid and do not like functions with a module, although there is nothing complicated about them. We have already touched on such things a little in the lesson. Geometric Plot Transformations. Since the modulus is non-negative, it expands as follows: 
, where "alpha" is some expression. In this case, , and our function should sign piecewise: 
But the fractions of both pieces have to be reduced by . The reduction, as in the previous example, will not go without consequences. The original function is not defined at the point because the denominator vanishes. Therefore, the system should additionally specify the condition , and make the first inequality strict: 
Now for a VERY USEFUL trick: before finalizing the task on a draft, it is beneficial to make a drawing (regardless of whether it is required by the condition or not). This will help, firstly, to immediately see the points of continuity and break points, and, secondly, it will 100% save you from errors when finding one-sided limits.
Let's do the trick. In accordance with our calculations, to the left of the point it is necessary to draw a fragment of the parabola (blue), and to the right - a piece of the parabola (red), while the function is not defined at the point itself: 
When in doubt, take a few "x" values, substitute them into the function 
(remembering that the module destroys a possible minus sign) and check the graph.
We investigate the function for continuity analytically:
1) The function is not defined at the point , so we can immediately say that it is not continuous at it.
2) Let us establish the nature of the discontinuity, for this we calculate one-sided limits: 
The one-sided limits are finite and different, which means that the function suffers a discontinuity of the 1st kind with a jump at the point . Once again, note that when finding the limits, it does not matter whether the function at the break point is defined or not.
Now it remains to transfer the drawing from the draft (it was made, as it were, with the help of research ;-)) and complete the task:
Answer: the function is continuous on the entire number line except for the point where it suffers a discontinuity of the first kind with a jump.
Sometimes it is required to additionally indicate the discontinuity jump. It is calculated elementarily - the left limit must be subtracted from the right limit: , that is, at the break point, our function jumped 2 units down (which the minus sign tells us about).
Example 3
Explore Function 
for continuity. Determine the nature of function breaks, if any. Make a drawing.
This is an example for self-solving, a sample solution at the end of the lesson.
Let's move on to the most popular and common version of the task, when the function consists of three pieces:
Example 4
Investigate the function for continuity and plot the function graph 
.
Solution: it is obvious that all three parts of the function are continuous on the corresponding intervals, so it remains to check only two "junction" points between the pieces. First, let's make a drawing on a draft, I commented on the construction technique in sufficient detail in the first part of the article. The only thing is to carefully follow our singular points: due to the inequality, the value belongs to the straight line (green dot), and due to the inequality, the value belongs to the parabola (red dot): 
Well, in principle, everything is clear =) It remains to draw up a decision. For each of the two "butt" points, we check 3 continuity conditions as a standard:
I) We examine the point for continuity
1) 
The one-sided limits are finite and different, which means that the function suffers a discontinuity of the 1st kind with a jump at the point .
Let us calculate the discontinuity jump as the difference between the right and left limits:
, that is, the chart jumped one unit up.
II) We examine the point for continuity
1) 
– the function is defined at the given point.
2) Find one-sided limits: 
– one-sided limits are finite and equal, so there is a common limit.
3) 
– the limit of a function at a point is equal to the value of this function at a given point.
At the final stage, we transfer the drawing to a clean copy, after which we put the final chord:
Answer: the function is continuous on the entire number line, except for the point where it suffers a discontinuity of the first kind with a jump.
Example 5
Investigate a function for continuity and build its graph 
.
This is an example for an independent solution, a short solution and an approximate sample of the problem at the end of the lesson.
One may get the impression that at one point the function must necessarily be continuous, and at another point there must necessarily be a discontinuity. In practice, this is not always the case. Try not to neglect the remaining examples - there will be several interesting and important features:
Example 6
Given a function 
. Investigate the function for continuity at points . Build a graph.
Solution: and again immediately execute the drawing on the draft: 
The peculiarity of this graph is that for the piecewise function is given by the equation of the abscissa axis. This area is shown here in green, and in a notebook it is usually highlighted in bold with a simple pencil. And, of course, do not forget about our sheep: the value refers to the tangent branch (red dot), and the value belongs to the straight line.
Everything is clear from the drawing - the function is continuous on the entire number line, it remains to draw up a solution that is brought to full automatism literally after 3-4 similar examples:
I) We examine the point for continuity
1) - the function is defined at a given point.
2) Calculate one-sided limits: 
, so there is a common limit.
Just for every firefighter, let me remind you of a trivial fact: the limit of a constant is equal to the constant itself. In this case, the limit of zero is equal to zero itself (the left-hand limit).
3) 
– the limit of a function at a point is equal to the value of this function at a given point.
Thus, a function is continuous at a point by the definition of a function being continuous at a point.
II) We examine the point for continuity
1) - the function is defined at a given point.
2) Find one-sided limits: 
And here - the limit of the unit is equal to the unit itself.
– there is a common limit.
3) 
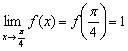– the limit of a function at a point is equal to the value of this function at a given point.
Thus, a function is continuous at a point by the definition of a function being continuous at a point.
As usual, after the study, we transfer our drawing to a clean copy.
Answer: the function is continuous at the points .
Please note that in the condition we were not asked anything about the study of the entire function for continuity, and it is considered good mathematical form to formulate precise and clear answer to the question posed. By the way, if according to the condition it is not required to build a graph, then you have every right not to build it (although later the teacher can force you to do this).
A small mathematical "patter" for an independent solution:
Example 7
Given a function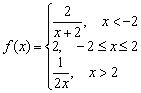 
. Investigate the function for continuity at points . Classify breakpoints, if any. Execute the drawing.
Try to correctly “pronounce” all the “words” =) And draw the graph more precisely, accuracy, it will not be superfluous everywhere ;-)
As you remember, I recommended that you immediately draw on a draft, but from time to time you come across such examples where you can’t immediately figure out what the graph looks like. Therefore, in a number of cases, it is advantageous to first find one-sided limits and only then, on the basis of the study, depict the branches. In the final two examples, we will also learn the technique of computing some one-sided limits:
Example 8
Investigate a function for continuity and build its schematic graph.
Solution: bad points are obvious: (turns the denominator of the exponent to zero) and (turns to zero the denominator of the entire fraction). It is not clear what the graph of this function looks like, which means that it is better to do research first.
